[數撚圍爐區] Representation Theory 好難 (46)
科大笑話王
1001 回覆
6 Like
5 Dislike
第 1 頁第 2 頁第 3 頁第 4 頁第 5 頁第 6 頁第 7 頁第 8 頁第 9 頁第 10 頁第 11 頁第 12 頁第 13 頁第 14 頁第 15 頁第 16 頁第 17 頁第 18 頁第 19 頁第 20 頁第 21 頁第 22 頁第 23 頁第 24 頁第 25 頁第 26 頁第 27 頁第 28 頁第 29 頁第 30 頁第 31 頁第 32 頁第 33 頁第 34 頁第 35 頁第 36 頁第 37 頁第 38 頁第 39 頁第 40 頁第 41 頁
加線呢個動作可以點表達?
仲有咩係點線面?
第五公理up乜春?
仲有咩係點線面?
第五公理up乜春?
仲有translation rotation呢D
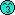
Theorem III.3.4. Suppose that Λ is a discrete valuation ring with residue field k and maximal
ideal m.
1. If P is a Λ[G]-module that is free of finite type4 over Λ, then P is a projective Λ[G]-module
if and only if P := P ⊗Λ k is a projective k[G]-module.
2. If P and P
0 are projective Λ[G]-modules, then P ' P
0 as Λ[G]-modules if and only if
P ⊗Λ k ' P
0 ⊗Λ k as k[G]-modules.
3. Suppose that the discrete valuation ring Λ is complete. (See problem VII.3.3.) If P is a
projective k[G]-module, then there exists a unique (up to isomorphism) projective Λ[G]-
module P such that P ' P ⊗Λ k.
ideal m.
1. If P is a Λ[G]-module that is free of finite type4 over Λ, then P is a projective Λ[G]-module
if and only if P := P ⊗Λ k is a projective k[G]-module.
2. If P and P
0 are projective Λ[G]-modules, then P ' P
0 as Λ[G]-modules if and only if
P ⊗Λ k ' P
0 ⊗Λ k as k[G]-modules.
3. Suppose that the discrete valuation ring Λ is complete. (See problem VII.3.3.) If P is a
projective k[G]-module, then there exists a unique (up to isomorphism) projective Λ[G]-
module P such that P ' P ⊗Λ k.